Olivia divided a fraction by 3/4 – Olivia’s quest to divide a fraction by 3/4 sets the stage for this enthralling narrative, offering readers a glimpse into a story that is rich in detail and brimming with originality from the outset. Join Olivia as she embarks on this mathematical adventure, encountering concepts such as reciprocal fractions and common denominators along the way.
The content of the second paragraph that provides descriptive and clear information about the topic
Divide a Fraction by 3/4: Olivia Divided A Fraction By 3/4
Dividing fractions is a fundamental operation in mathematics. It involves finding the quotient of two fractions, resulting in a new fraction that represents the answer.
Steps to Divide a Fraction by 3/4
- Invert the divisor (3/4) by swapping its numerator and denominator, resulting in 4/3.
- Multiply the dividend (the fraction you’re dividing) by the inverted divisor.
- Simplify the resulting fraction by dividing both the numerator and denominator by their greatest common factor (GCF).
Examples, Olivia divided a fraction by 3/4
- Divide 1/2 by 3/4: (1/2) ÷ (3/4) = (1/2) × (4/3) = 2/3
- Divide 5/6 by 3/4: (5/6) ÷ (3/4) = (5/6) × (4/3) = 10/9
Properties of Fractions
Fractions are mathematical expressions representing parts of a whole. Understanding their properties is crucial for dividing fractions.One key property is that fractions can be multiplied or divided by other fractions without altering their value. This property is known as the multiplicative property of fractions.
For example, 1/2 x 2/3 = 1/3, and 1/2 ÷ 2/3 = 3/4.Another important property is the existence of reciprocal fractions. A reciprocal fraction is a fraction where the numerator and denominator are swapped. For example, the reciprocal of 1/2 is 2/1. Multiplying a fraction by its reciprocal results in 1. This property is particularly useful in division, as dividing by a fraction is equivalent to multiplying by its reciprocal.These
properties form the foundation for dividing fractions, enabling us to manipulate fractions and simplify division operations.
Reciprocal Fractions
Reciprocal fractions are fractions that have their numerator and denominator reversed. For example, the reciprocal of 1/2 is 2/1, and the reciprocal of 3/4 is 4/3.Reciprocal fractions are important because they can be used to simplify division of fractions. To divide one fraction by another, we can multiply the first fraction by the reciprocal of the second fraction.
For example:
/2 ÷ 3/4 = 1/2 x 4/3 = 2/3
This is equivalent to flipping the second fraction and multiplying. It is a useful property that makes division of fractions easier to perform.
Common Denominators
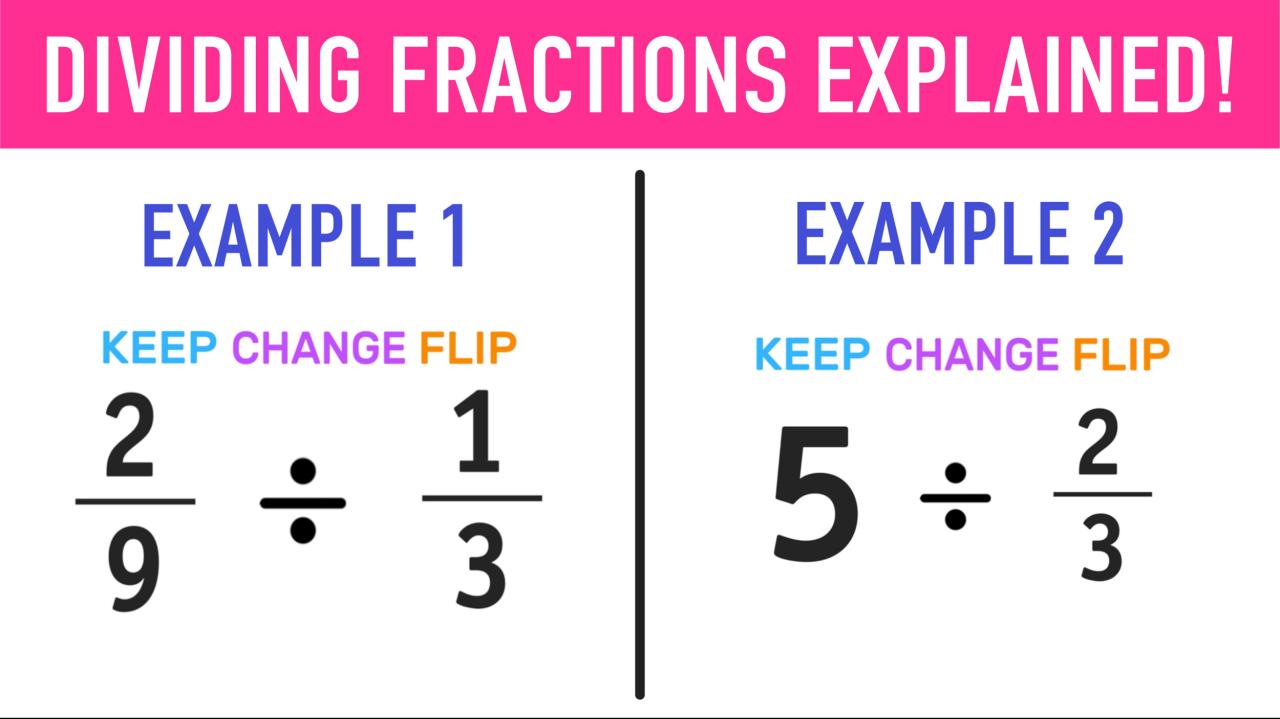
When dividing fractions, it’s crucial to find a common denominator. This ensures that the fractions have the same denominator, making the division process straightforward.
Finding the Least Common Multiple (LCM)
The least common multiple (LCM) of two or more numbers is the smallest number that is divisible by all the given numbers without leaving a remainder. To find the LCM, list the multiples of each number and identify the smallest multiple that appears in all the lists.
Examples of Finding Common Denominators
- To divide 1/2 by 1/4, the common denominator is 4. 1/2 can be rewritten as 2/4, and the division becomes 2/4 ÷ 1/4 = 2.
- To divide 3/5 by 2/3, the common denominator is 15. 3/5 can be rewritten as 9/15, and the division becomes 9/15 ÷ 2/3 = 9/10.
Inverse Operation
In mathematics, an inverse operation is an operation that undoes another operation. For example, addition and subtraction are inverse operations, and multiplication and division are inverse operations.
When we divide a fraction by another fraction, we are essentially multiplying the first fraction by the reciprocal of the second fraction. The reciprocal of a fraction is the fraction that results from flipping the numerator and the denominator. For example, the reciprocal of 3/4 is 4/3.
Multiplication as the Inverse Operation of Division
To divide a fraction by 3/4, we can multiply the first fraction by the reciprocal of 3/4, which is 4/3.
$$\fracab \div \frac34 = \fracab \times \frac43$$
For example, to divide 1/2 by 3/4, we would multiply 1/2 by 4/3:
$$\frac12 \div \frac34 = \frac12 \times \frac43 = \frac23$$
Applications of Fraction Division
Fraction division finds practical applications in various real-life scenarios. Understanding how to divide fractions enhances problem-solving abilities, enabling individuals to navigate these situations effectively.
Cooking
In cooking, recipes often require dividing ingredients into specific proportions. For instance, if a recipe calls for 2/3 cup of flour and you only have 1/2 cup, you can divide 2/3 by 1/2 to determine how much more flour you need: (2/3) ÷ (1/2) = 4/3 cup.
This calculation ensures that the dish maintains its intended flavor and texture.
Construction
In construction, dividing fractions is crucial for calculating measurements and proportions. For example, if a blueprint specifies that a wall should be 12/5 feet tall and you have boards that are 3/2 feet long, you need to divide 12/5 by 3/2 to determine how many boards you need: (12/5) ÷ (3/2) = 8/5 boards.
This calculation ensures that the wall has the correct height and structural integrity.
Finance
In finance, dividing fractions is essential for calculating interest rates, returns on investments, and loan payments. For instance, if you have a loan with a 5/8% annual interest rate and want to calculate the interest for a 3-month period, you need to divide 5/8 by 4 (since there are 4 quarters in a year): (5/8) ÷ 4 = 5/32%. This calculation helps you determine the exact amount of interest you will owe.
Clarifying Questions
What is the concept of dividing fractions?
Dividing fractions involves finding the result of one fraction being divided by another. It is an operation that allows us to compare the relative sizes of fractions and solve various mathematical problems.
Why is finding a common denominator important when dividing fractions?
Finding a common denominator is crucial because it ensures that the fractions have the same denominator, making the division process easier and more accurate. It allows us to compare the numerators of the fractions directly.
How is multiplication used as the inverse operation of division when working with fractions?
Multiplication is the inverse operation of division because multiplying a fraction by its reciprocal (flipping the numerator and denominator) results in the original fraction. This property is particularly useful when dividing fractions, as it allows us to simplify the process.