Regents exam questions s.id.b.6 regression 1 – Embark on a journey through Regents Exam Questions: S.ID.B.6 Regression 1, a comprehensive guide that empowers students with the knowledge and strategies to excel in this challenging subject. This meticulously crafted resource provides an in-depth exploration of regression analysis, equipping readers with the tools necessary to navigate its complexities and achieve success.

Delve into the intricacies of regression questions, master data analysis techniques, and discover the practical applications of this powerful statistical tool. Prepare to conquer the Regents Exam with confidence and emerge as a true master of regression analysis.

Regents Exam Questions

S.ID.B.6 Regression 1

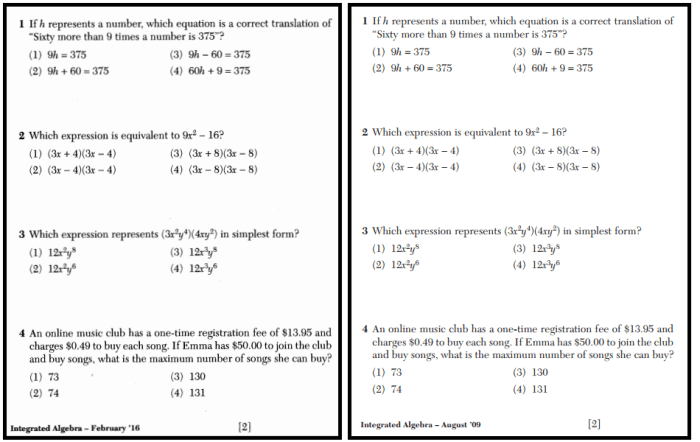
Regents Exam Questions for S.ID.B.6 Regression 1 are designed to assess students’ understanding of linear regression concepts and their ability to apply regression techniques to real-world data. These questions evaluate students’ proficiency in data analysis, interpretation, and the construction and interpretation of regression models.

Types of Regression Questions

• Scatterplot Analysis:Questions that require students to analyze a scatterplot and identify the type of relationship between two variables (positive, negative, or no relationship).
• Regression Line Determination:Questions that ask students to determine the equation of the regression line for a given data set.
• Data Interpretation:Questions that require students to interpret the slope and y-intercept of a regression line and make predictions based on the model.
• Residual Analysis:Questions that assess students’ ability to identify outliers and assess the goodness of fit of a regression model.

Data Analysis and Interpretation

Data analysis and interpretation are crucial in regression questions. Students must be able to examine scatterplots, identify patterns, and determine the strength and direction of the relationship between variables. They should also be able to identify outliers and assess the reliability of the regression model.

Regression Line and Equation

The regression line is a straight line that best fits the data points in a scatterplot. The equation of the regression line can be determined using various methods, including the least squares method. Students should be able to calculate the slope and y-intercept of the regression line and use the equation to make predictions.

Correlation and Causation

Correlation refers to the strength and direction of the relationship between two variables, while causation implies that one variable causes the change in another. In regression analysis, it is important to distinguish between correlation and causation. Students should be able to identify when a relationship is correlational or causal and avoid making causal inferences based solely on correlation.

Applications of Regression

Regression analysis has numerous practical applications in various fields, including:

• Predicting future values based on historical data (e.g., predicting sales based on advertising expenditure).
• Identifying relationships between variables (e.g., studying the relationship between student attendance and academic performance).
• Making decisions based on data-driven insights (e.g., determining the optimal price point for a product based on market research).

Tips for Success: Regents Exam Questions S.id.b.6 Regression 1

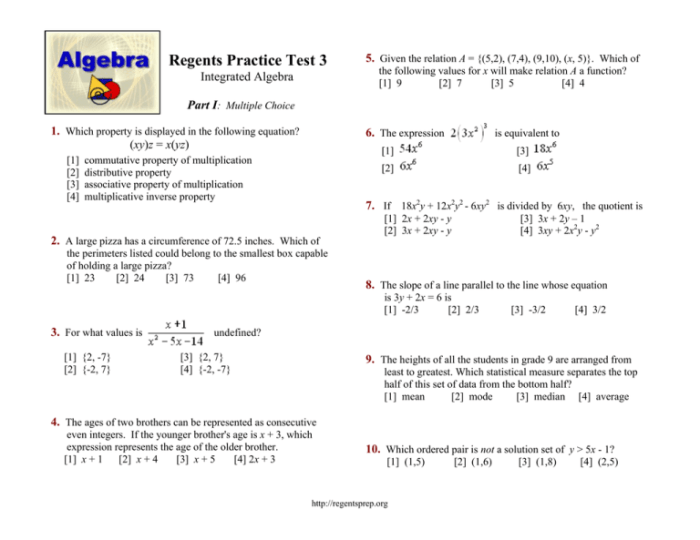
• Understand the concepts of regression and its applications.
• Practice analyzing scatterplots and identifying relationships between variables.
• Learn the methods for determining the regression line equation.
• Develop skills in data interpretation and prediction using regression models.
• Distinguish between correlation and causation in regression analysis.
• Manage time effectively during the exam and focus on answering questions that you are confident in.

User Queries

What is the purpose of regression analysis?

Regression analysis is a statistical technique used to investigate the relationship between a dependent variable and one or more independent variables. It allows us to predict the value of the dependent variable based on the values of the independent variables.

How do I determine the regression line and equation?

The regression line is a straight line that best fits the data points. The regression equation is the mathematical equation that represents the regression line. To determine the regression line and equation, we use a statistical technique called least squares regression.

What is the difference between correlation and causation?

Correlation measures the strength and direction of a linear relationship between two variables. Causation implies that one variable directly causes changes in another variable. Correlation does not imply causation, and it is important to be cautious when interpreting correlations.